Think of a square dotted with points. Now imagine the smallest triangle that could be made by connecting three of those points. That’s the Heilbronn triangle problem in a nutshell.
“The problem is very easy to state and can sound frivolous,” says Cosmin Pohoata, a theoretical mathematician and Emory assistant professor of mathematics. “When I have conversations with non-math friends, they often ask me why we should study problems like this. The beauty of them is that they are often more complex than they seem. They can have unexpected connections that open new doors for understanding all sorts of phenomena.”
Pohoata and two MIT graduate students, Alex Cohen and Dimitrii Zakharov, recently opened some of those new doors. They completed a new proof for the Heilbronn triangle problem that shows that the smallest triangle in a confined space is much smaller than was previously realized, breaking a record that stood for 40 years.
Their proof, available online, is submitted to the Journal of the American Mathematical Society and is already making waves in the math world.
“I think it’s a stunning result,” Anthony Carbery, a mathematician at the University of Edinburgh, told Qanta Magazine. And Thomas Bloom of the University of Oxford told Qanta that he expects the new proof to “prompt a renaissance” of progress on the triangle problem.
“What makes the proof special to me,” Pohoata says, “is the way that we connected the triangle problem to different areas of math. In particular, harmonic analysis, the study of how waves interact with one another and projection theory, or the behavior of fractals under projections.”
The making of a mathematician
Pohoata loved math from the time he was a small child growing up in Romania. He cites an elementary school teacher, who encouraged his love for numbers and patterns, as one key influence.
He began competing in International Mathematical Olympiads (IMO) during middle school. Romania is the original home of the IMO, which dates back to 1959, making it the oldest of the International Science Olympiads. Today more than 100 countries compete in the annual event.
“I thought I knew a lot about math because the problems in class had been so easy for me,” Pohoata recalls. “When I started competing in the Olympiads, I began to realize how little I knew and how much math was out there to learn.”
He began to think about math as a career. “It’s quite fun to get to think about problems that interest you,” he says.
Pohoata attended Princeton as an undergraduate, got his PhD at the California Institute of Technology and taught at Yale before joining Emory this fall.
Patterns in points and lines
As a theoretical mathematician, Pohoata focuses his research on three specialized fields: discrete geometry, additive number theory and extremal combinatorics.
Extremal combinatorics examines how large or small finite objects such as graphs can be, if placed under certain restrictions. For centuries, it seemed like an esoteric endeavor.
“The breadcrumbs to extremal combinatorics trace back to ancient Greece,” Pohoata says, “but the field didn’t really come alive until the late 20th century with the rise of computers and the internet. Graphs are at the heart of many things to do with computer science and the internet.”
Facebook “friend” networks, for example, are large collections of data that are essentially graphs. “You can think of people in the world as points on paper and then draw arrows connecting the ones who are friends,” Pohoata explains. “Then you can look at basic questions underlying these structures. If you have at least seven points do you always have triangles? When do you see a big cluster of connected vertices? Are there areas of the world that are less connected than others?”
Real-life problems, like how to make algorithms run faster, fuel interest in studying problems about graphs and related areas.
“As a theorist, I’m driven simply by the math behind shapes and the beauty of them,” Pohoata says, “but I do get excited when I hear that some math breakthrough has been used in a cool way to help with a practical problem.”
The human side of math
“I wasn’t interested in the history of math when I first started out,” Pohoata says. “Why learn the progression of results if you have the latest result?”
But when he taught an introductory course to number theory, it forced him to look more closely at the history of the greats and trace the chronology of events.
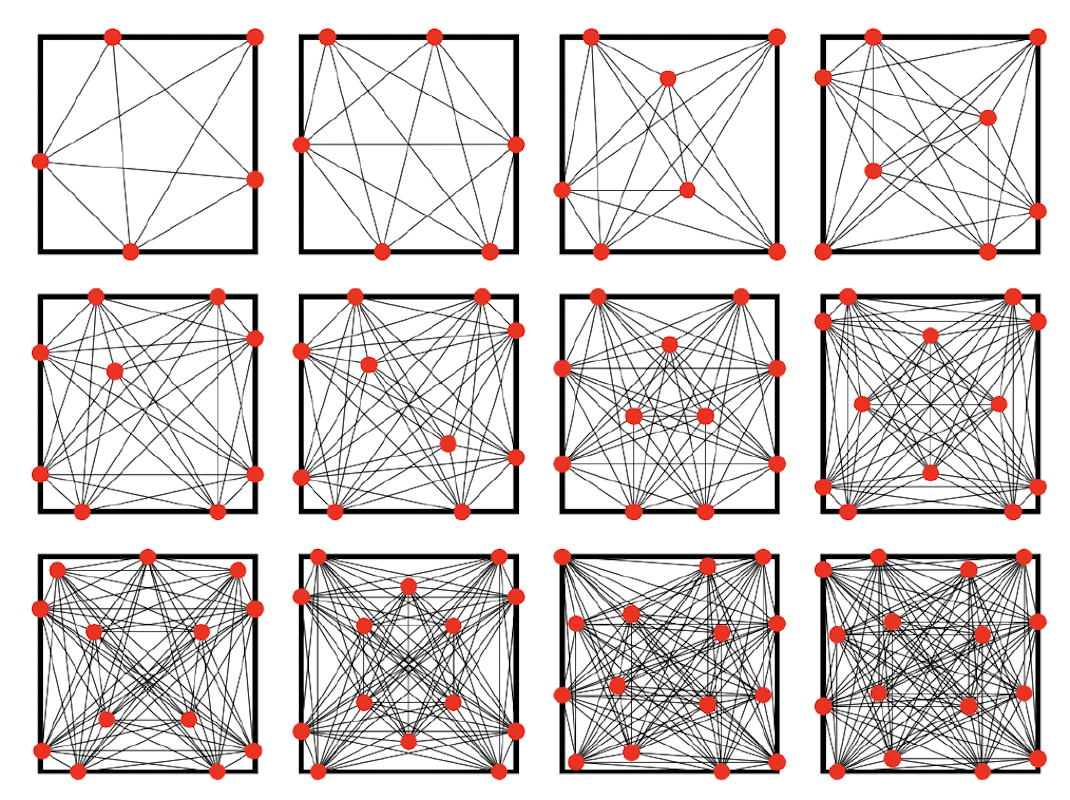
A graphic representation of the Heilbronn triangle problem.
Pohoata’s interest in the Heilbronn triangle problem inspired him to delve deeper into the work of Klaus Roth, a German-British mathematician who won math’s highest honor, the Fields Medal. “Roth does elegant math that has inspired a lot of activity,” Pohoata says.
In 1951, Roth developed a strategy for finding the smallest possible triangle within the parameters of the Heilbronn triangle problem, or its so-called “upper bound.”
Austrian mathematician Wolfgang Schmidt pushed the upper bound further in a paper published in 1972. That inspired Roth to jump back into the game. Roth further improved the result by Schmidt, just a few months after Schmidt’s breakthrough.
A tiny problem
“Roth and Schmidt had a kind of rivalry to see who could come up with the best recipe to find even smaller triangles,” Pohoata says. “They were writing beautiful papers, improving on each other’s work. I learned a lot by studying them.”
In 1980, a trio of mathematicians — Komlos, Pintz and Szemeredi — pushed the envelope even further, finding a new upper bound to the Heilbronn triangle problem.
While the problem in its simplest form can be thought of as dots and lines drawn on paper, the mathematicians are working with triangles far too tiny to be “seen” without special tools.
“You can think of these triangles as microscopic,” Pohoata says. “We’re talking about billions of points crammed within a confined space.”
Just as scientists keep making improvements in microscopy to get an ever more detailed view of the tiniest parts of a living system or of distant galaxies imperceptible to human eyes on Earth, theoretical mathematicians create tools to get closer and sharper views of the math underlying the universe and everything in it.
Making connections
Pohoata had pondered the Heilbronn triangle problem for several months with Zakharov. He met Cohen last year in a chance encounter at MIT, where he had traveled to give a presentation.
“When math people get together, they like to talk about recent problems on their minds,” he says. “We were excited to learn that we were taking similar approaches to the Heilbronn triangle problem. And that we were all stuck in the same place.”
The trio decided to join forces. Unlike many of their math heroes of the past, who communicated across distances by letter, they exchanged ideas in real time through Zoom and the Discord instant-messaging platform.
“Math research is becoming more of a social experience as the world has become more connected,” Pohoata says. “Technology facilitates collaboration.”
Rather than a single, euphoric eureka moment, he describes the process of creating their 40-page proof as a series of smaller insights.
“There are many moving pieces to this proof and each one had to come together,” Pohoata explains. “There was a lot of going back and forth to get all the pieces to fit. You can think of it like putting together a really complex Lego structure.”
Ultimately, their breakthrough revealed new connections between the Heilbronn triangle problem and other areas of mathematics, including harmonic analysis and fractals — figures that are similar and keep repeating one another at smaller and smaller scales.
Pohoata and his two MIT colleagues are continuing to work on explaining this web of connections in more detail.
“It’s a very rich area, to study tiny, small-scale shapes and uncover what the math hidden there can tell us,” Pohoata says. “What makes math fascinating is that it’s the language for how things work in the world.”
Listen to Cosmin Pohoata give a talk about the new proof.

